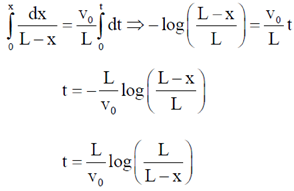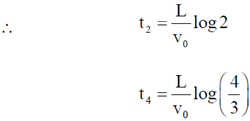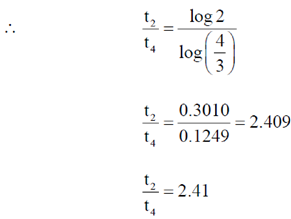1. The dispersion relation for electromagnetic waves travelling in a plasma is given as where c and 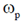are constants. In this plasma, the group velocity is

(a) proportional to but not equal to the phase velocity

(b) inversely proportional to the phase velocity

(c) equal to the phase velocity

(d) a constant

Ans. (b)

Sol. From the definition of group velocity,

Given that,

On differentiating Eq. (ii) w.r.t. k, we get

Putting the value from Eq. (iii) into Eq. (i), we get

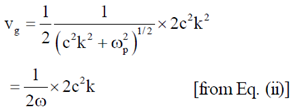
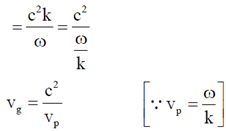
where, v_p = phase velocity

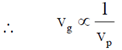
where, v_p = phase velocity

Hence, group velocity (v_p) is inversely proportional to the phase velocity (v_p).

2. Consider the following circuit with two identical Si diodes. The input ac voltage waveform has the peak voltage V_P = 2V, as shown

The voltage waveform across PQ will be represented by

(a)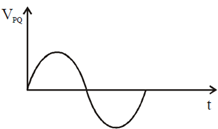

(b)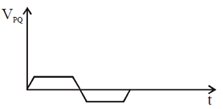

(c)

(d)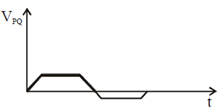

Ans. (c)

Sol. We know that the voltage drop across each Si diode is

V₀ = 0.7 V

Case I For forward blased,

By using formula, V_out = V_P – 2V₀

[given V_p = 2V]

V_out = 2 – 2 1.4

V_out = 0.6 V ... (i)

Case II For reversed biased,

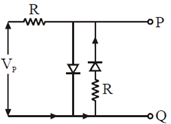
By using formula, V_out = V_P – V₀

V_out = 2 – 0.7 = 1.3 V ... (ii)

From Eqs. (i) and (ii), It is clear that, the voltage waveform across PQ will be represented by

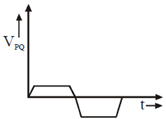
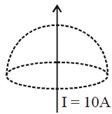
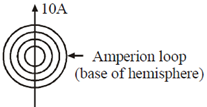
3. A current I = 10A flows in an infinitely long wire along the axis of a hemisphere (see figure). The value of 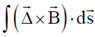over the hemispherical surface as shown in the figure is

(a)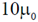

(b)

(c) 0

(d)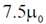

Ans. (a)

Sol. From Ampere's circuital law in integral form,

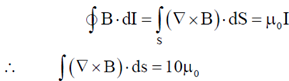
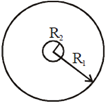
4. Consider two, single turn, co-planar, concentric coils of radii R₁ and R₂ with R₁ >> R₂. The mutual inductance between the two coils is proportional to

(a) R₁/R₂

(b) R₂/R₁

(c)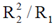

(d)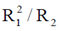

Ans. (c)

Sol. Suppose the current flow in outer ring is I₁.

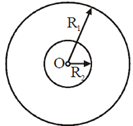
Then, we know that the magnetic field at the centre of the ring due to I₁ is

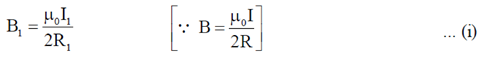
Since R₁ >> R₂, we approximate the magnetic field through the entire inner coil be B₁.

Hence, the flux through the inner coil is

Here, A₂ = area of inner ring

On putting the values from Eqs. (i) and (iii) into Eq. (ii), we get

Thus, the mutual inductance is given by

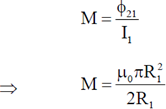
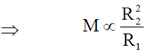
5. If the Boolean function Z = PQ + PQR + PQRS + PQRST + PQRSTU, then 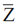is

(a)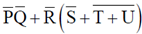

(b)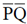

(c)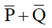

(d)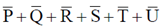

Ans. (c)

Sol. For Boolean algebra, 1 + A = 1

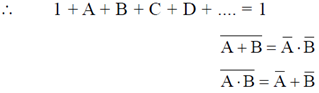
So, according to question,

Z = PQ + PQR + PQRS + PQRST + PQRSTU

= PQ(1 + R + RS + RST + RSTU)

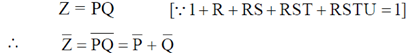
6. Shown in the figure is a combination of logic states. The output values at P and Q are correctly represented by which of the following?

(a) 0 0

(b) 1 1

(c) 0 1

(d) 1 0

Ans. (c)

Sol.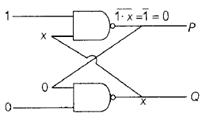

Let output at Q is x,

i.e. x = Q ... (i)

Now, from first AND gate operation,

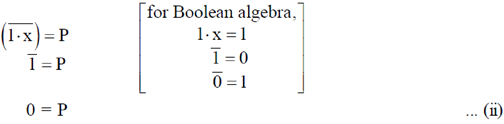
From second NAND gate operation,

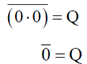
1 = Q ... (iii)

Hence, P = 0, Q = 1

7. Which of the following is due to inhomogeneous refractive index of earth's atmosphere?

(a) Red colour of the evening Sun

(b) Blue colour of the sky

(c) Oval shape of the evening Sun

(d) Large apparent size of the evening Sun

Ans. (c)

Sol. The rays of light from upper and lower edges of sun bend unequally due to in homogeneous refractive index of earth's atmosphere. Due to unequal bending of light, the image of sun appears oval and larger in size.

8. For the three matrices given below, which one of the choices is correct?

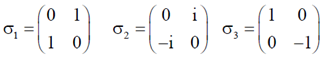
(a)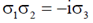

(b)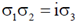

(c)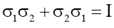

(d)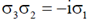

Ans. (a)

Sol.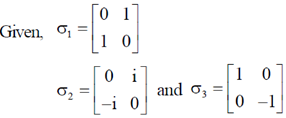

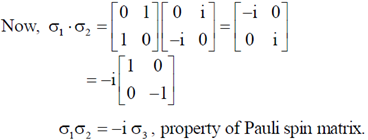
9. A plane in a cubic lattice makes intercepts of a, a/2 and 2a/3 with the three crystallographic axes, respectively. The Miller indices for this plane are

(a) (2 4 3)

(b) (3 4 2)

(c) (6 3 4)

(d) (1 2 3)

Ans. (a)

Sol.

Multiply from LCM for denominator,

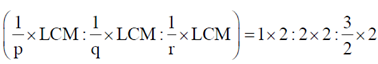
Hence, the Miller indices of the plane = (2, 4, 3).

10. Which one of the following schematic curves best represents the variation of conductivity of a metal with temperature T?

(a)

(b)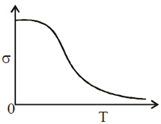

(c)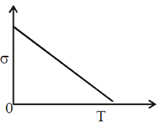

(d)

Ans. (b)

Sol. We know that, conductivity of metal (conductor),

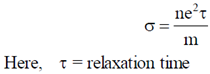
n = number of electrons

e = charge of electron

m = mass of electron

We also know that relaxation time decreases with increase in temperature, hence conductivity of metals decreases rapidly with temperature, but in relaxation time conductivity is same.

11. Consider an inertial frame S' moving at speed c/2 away from another inertial frame S along the common x – x' axis, where c is the speed of light. As observed from S', a particle is moving with speed c/2 in the y' direction, as shown in the figure. The speed of the particle as seen from S is:

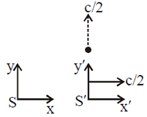
(a)

(b) c/2

(c)

(d)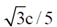

Ans. (c)

Sol.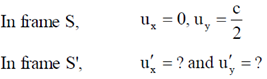

By the Lorentz transformation of velocity,

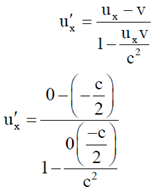
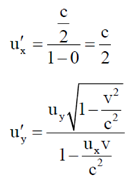
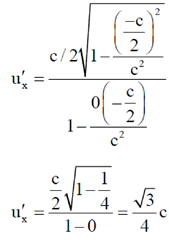
So, resultant velocity is

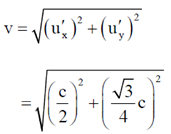
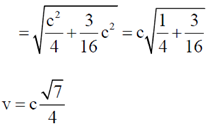
12. Consider a uniform thin circular disk of radius R and mass M. A concentric square of side R/2 is cut out from the disk (see figure). What is the moment of inertia of the resultant disk about an axis passing through the centre of the disk and perpendicular to it?

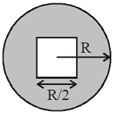
(a)

(b)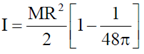

(c)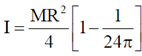

(d)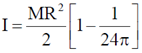

Ans. (b)

Sol. We know that, moment of inertia of disc,

We also know that, moment of inertia of square,

Here, M' = mass of square

 

On putting the values from Eqs. (iii) and (iv) into Eq. (ii), we get

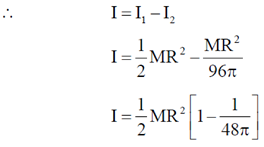
13. Consider a system of N particles obeying classical statistics, each of which can have an energy 0 or E. The system is in thermal contact with a reservoir maintained at a temperature T. Let k denote the Boltzmann constant. Which one of the following statements regarding the total energy U and the heat capacity C of the system is correct?

(a)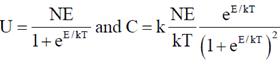

(b)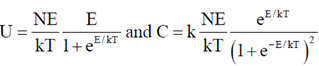

(c)

(d)

Ans. (d)

Sol. We know that, from Boltzmann's distribution,

But N₀ + N_i = N

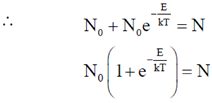
From Eqs. (i) and (ii), we get

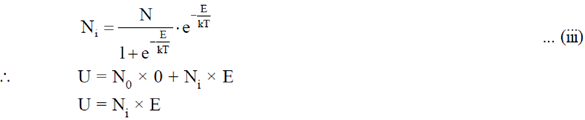
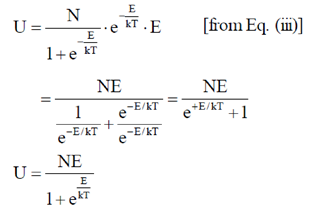
14. The integral of the vector 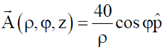(standard notation for cylindrical coordinates is used) over the volume of a cylinder of height L and radius R₀ is

(a)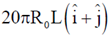

(b) 0

(c)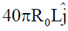

(d)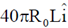

Ans. (d)

Sol.

We know that in cylindrical coordinates system,

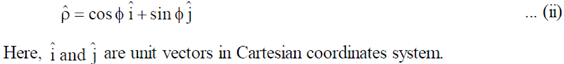
From Eqs. (i) and (ii), we get

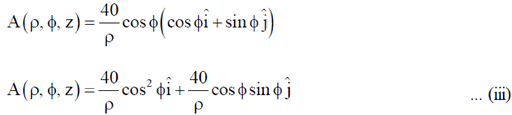
On taking volume integral of Eq. (iii),

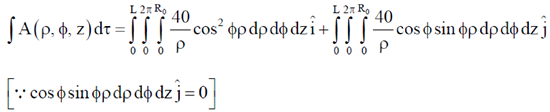
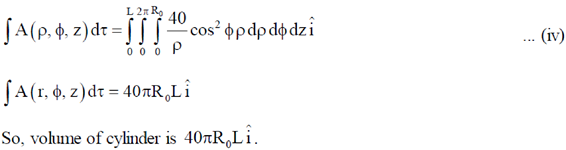
15. Consider Rydberg (hydrogen-like) atoms in a highly excited state with n around 300. The wavelength of radiation coming out of these atoms for transitions to the adjacent states lies in the range:

(a)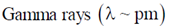

(b)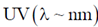

(c)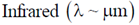

(d)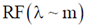

Ans. (d)

Sol. We know that for hydrogen like atom,

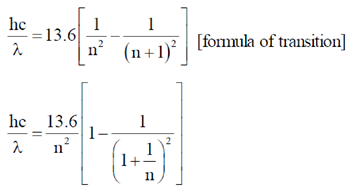
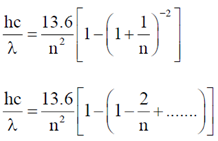
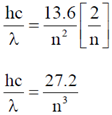
Given, n = 300

From Eq. (i), we get

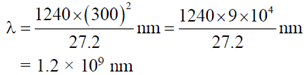
16. A uniform rigid meter-scale is held horizontally with one of its end at the edge of a table and the other supported by hand. Some coins of negligible mass are kept on the meter scale as shown in the figure.

As the hand supporting the scale is removed, the scale starts rotating about its edge on the table and the coins start moving. If a photograph of the rotating scale is taken soon after, it will look closest to

(a)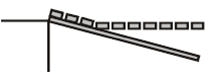

(b)

(c)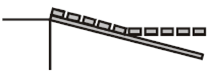

(d)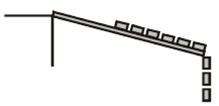

Ans. (b)

Sol. Angular acceleration given by

Putting these values from Eqs. (ii) and (iii) into Eq. (i), we get

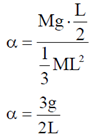
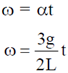
So, coin be in contact with rod there after coin will get detached from rod.

We know that, angular velocity,

17. Consider two identical, finite, isolated systems of constant heat capacity C at temperature T₁ and T₂, (T₁ > T₂). Work performed by the engine will be maximum (= W_max) if the process is reversible (equivalently, the entropy change of the entire system is zero), the value of W_max is

(a) C(T₁ – T₂)

(b) C(T₁ – T₂)/2

(c)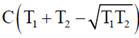

(d)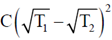

Ans. (d)

Sol. Consider unit mass substance (m = 1).

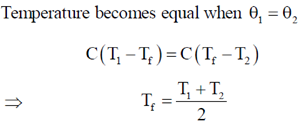
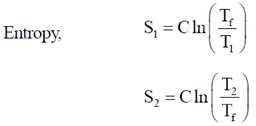
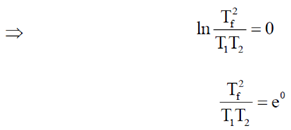
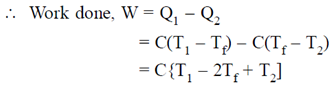
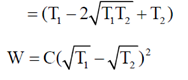
18. A white dwarf star has volume V and contains N electrons so that the density of electron is 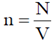. Taking the temperature of the star to be 0 K, the average energy per electron in the star is 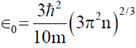, where m is the mass of the electron. The electronic pressure in the star is

(a)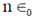

(b)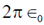

(c)

(d)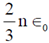

Ans. (d)

Sol.

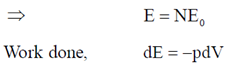
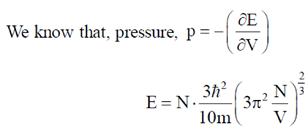
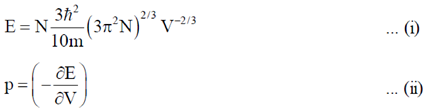
On differential Eq. (i) w.r.t. V and put in Eq. (ii), we get

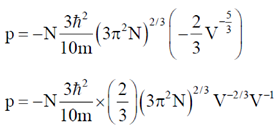
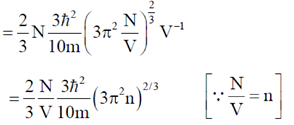
19. A pendulum is made of a massless string of length L and a small bob of negligible size and mass m. It is released making an angle 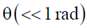from the vertical. When passing through the vertical, the string slips a bit from the pivot so that its length increases by a small amount 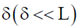in negligible time. If it swings up to angle on the other side before starting to swing back, then to a good approximation which of the following expression is correct?

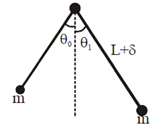
(a)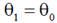

(b)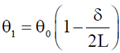

(c)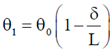

(d)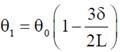

Ans. (b)

Sol.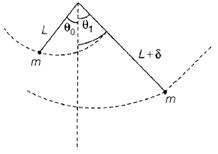

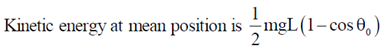
By the law of conservation of energy,

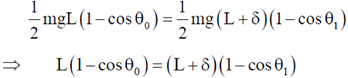
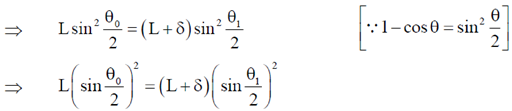
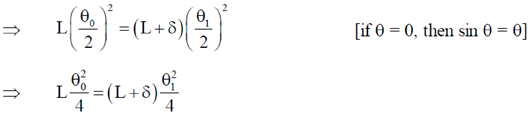
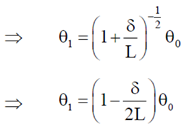
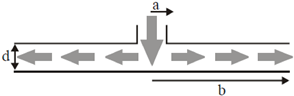
20. To demonstrate Bernoulli's principle, an instructor arrange two circular horizontal plates of radii b each with distance d(d << b) between them (see figure). The upper plate has a hole of radius a in the middle. On blowing air at a speed v₀ through the hole so that the flow rate of air is 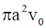it is seen that the lower plate does not fall. if the density of air is 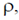the upward force on the lower plate is well approximated by the formula (assume that the region with r < a does not contribute to the upward force and the speed of air at the edges is negligible)

(a)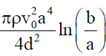

(b)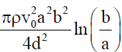

(c)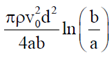

(d)

Ans. (a)

Sol. Let v₀ and v₁ are velocity at r = a and r = r

Apply continuity equation,

Pressure difference,

 

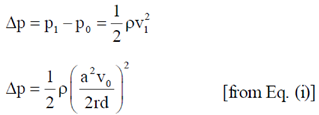
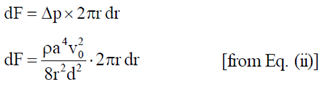
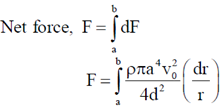
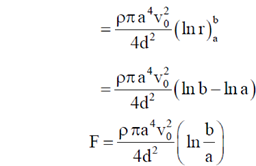
21. KCl has the NaCl type structure which is fcc with two-atom basis, one at (0, 0, 0) and the other at 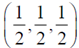. Assume that the atomic form factors of K⁺ and Cl^– are identical. In an x-ray diffraction experiment on KCl, which of the following (h k l) peaks will be observed?

(a) (1 0 0)

(b) (1 1 0)

(c) (1 1 1)

(d) (2 0 0)

Ans. (d)

Sol. From Bragg's diffraction condition,

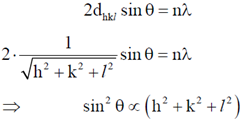
For fcc lattice,

peaks observed, if

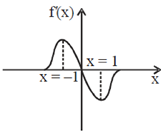
22. Which one of the following graphs represents the derivative 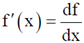of the function 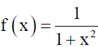most closely (graphs are schematic and not drawn to scale)?

(a)

(b)

(c)

(d)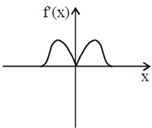

Ans. (a)

Sol.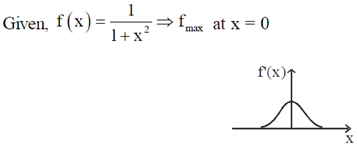

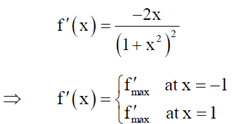
23. In the radiation emitted by a black body, the ratio of the spectral densities at frequencies 2v and v will vary with v as

(a)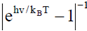

(b)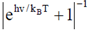

(c)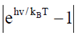

(d)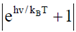

Ans. (b)

Sol.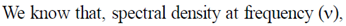

n dividing Eq. (ii) by Eq. (i), we get

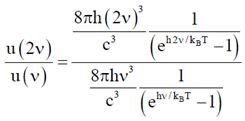
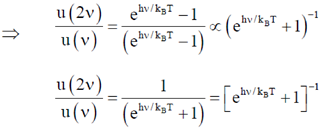
24. Consider a thin insulator coated conducting wire carrying current I. It is now wound once around an insulating thin disc of radius R to bring the wire back on the same side, as shown in the figure. The magnetic field at the centre of the disc is equal to:

(a)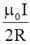

(b)

(c)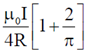

(d)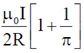

Ans. (b)

Sol.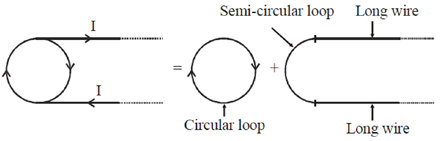

Total magnetic field,

B = B_{circular loop} + B_{semi-circular loop} + 2B_{long wire}

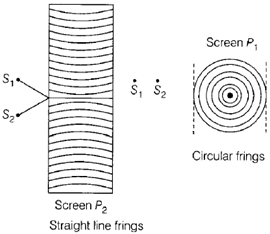
25. Consider two coherent point sources (S₁ and S₂) separated by a small distance along a vertical line and two screen P₁ and P₂ placed as shown in Figure. Which one of the choices represents the shapes of the interference fringes at the central regions on the screens?

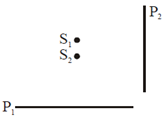
(a) Circular on P₁ and straight lines on P₂

(b) Circular on P₁ and circular on P₂

(c) Straight lines on P₁ and straight lines on P₂

(d) Straight lines on P₁ and circular on P₂

Ans. (a)

Sol. From Young's double slit experiment,

26. The electric field of an electromagnetic wave is given by

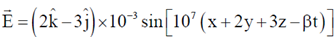
The value of 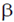is (c is the speed of light)

(a)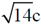

(b)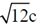

(c)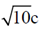

(d)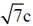

Ans. (a)

Sol. Given that, the electric field of an electromagnetic wave is

 

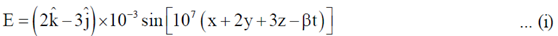
We know that, general equation of electromagnetic wave is

On comparing Eqs. (i) and (ii), we get

 

 

 

We also know that,

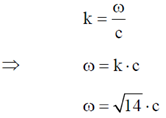
From Eqs. (i) and (ii), we get

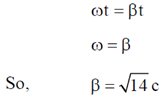
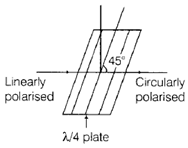
27. Unpolarized light is incident on a combination of a polarizer, a 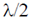plate and a 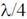plate kept one after the other. What will be the output polarization for the following configurations?

Configuration 1: Axes of the polarizer, the 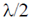plate and the 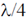plate are all parallel to each other.

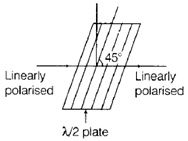
Configuration 2: The 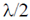plate is rotated by 45º with respect to configuration 1

Configuration 3: The 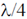plate is rotated by 45º with respect to configuration 1

(a) Linear for configuration 1, linear for configuration 2, circular for configuration 3

(b) Linear for configuration 1, circular for configuration 2, circular for configuration 3

(c) Circular for configuration 1, circular for configuration 2, circular for configuration 3

(d) Circular for configuration 1, linear for configuration 2, circular for configuration 3

Ans. (a)

Sol.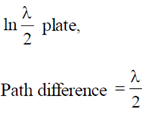

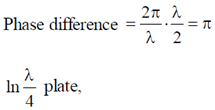
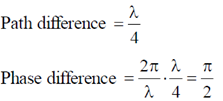
Configuration 1

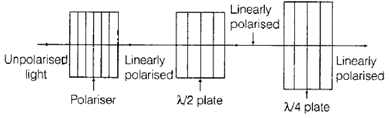
Configuration 2

Configuration 3

28. For the Fourier series of the following function of period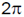

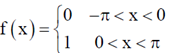
the ratio (to the nearest integer) of the Fourier coefficients of the first and the third harmonic is

(a) 1

(b) 2

(c) 3

(d) 6

Ans. (c)

Sol.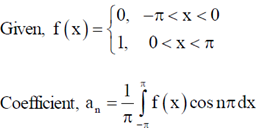

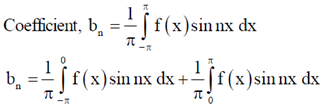
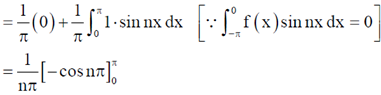
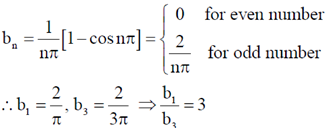
29. An n-p-n transistor is connected in a circuit as shown in the figure. If I_C = 1mA, = 50, V_BE = 0.7V, and the current through R₂ is 10I_B, where I_B is the base current. Then the ratio R₁/R₂ is

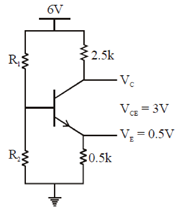
(a) 0.375

(b) 0.25

(c) 0.5

(d) 0.275

Ans. (d)

Sol.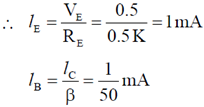

6 – R₁ × 11l_B – R₂ × 10 l_B = 0

11R₁ + 10R₂ = 300 ... (i)

and 6 – 11l_B × R₁ – 0.7 – 0.5 = 0 ... (ii)

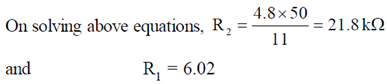
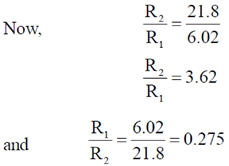
30. A rectangular loop of dimension L and width w moves with a constant velocity v away from an infinitely long straight wire carrying a current I in the plane of the loop as shown in the figure below. Let R be the resistance of the loop. What is the current in the loop at the instant the near-side is at a distance r from the wire

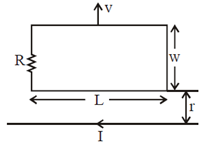
(a)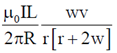

(b)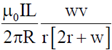

(c)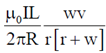

(d)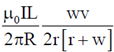

Ans. (c)

Sol.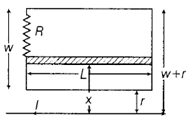

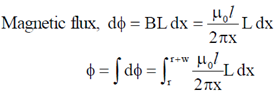
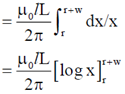
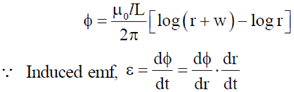
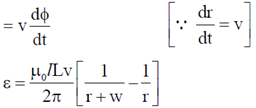
31. A photon of frequency 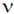strikes an electron of mass m initially at rest. After scattering at an angle 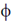, the photon loses half of its energy. If the electron recoils at an angle 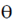, which of the following is (are) true?

(a)

(b)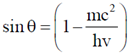

(c) The ratio of the magnitudes of momenta of the recoiled electron and scattered photon is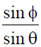

(d) Change in photon wavelength is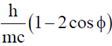

Ans. (a, c)

Sol.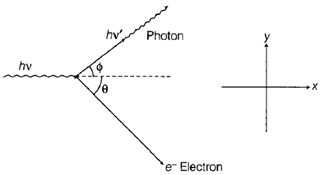

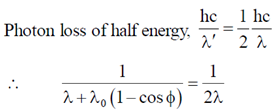
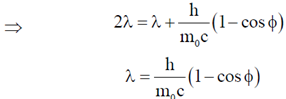
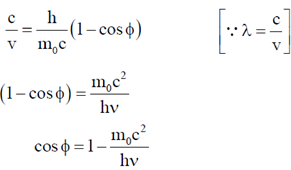
Conserving linear momentum in x-direction,

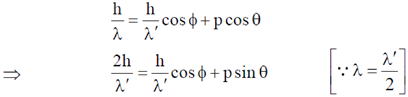
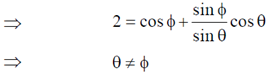
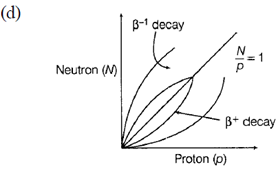
32. For an atomic nucleus with atomic number Z and mass number A, which of the following is (are) correct?

(a) Nuclear matter and nuclear charge are distributed identically in the nuclear volume

(b) Nuclei with Z > 83 and A > 209 emit -radiation

(c) The surface contribution to the binding energy is proportional to A^2/3

(d) -decay occurs when the proton to neutron ratio is large, but not when it is small

Ans. (b, c)

Sol. Nuclear matter and nuclear charge are distributed identically.

(b) Nuclei Z > 83 < A > 209, i.e. , higher nucleus emits -particles to gain stability as shown by binding energy curve.

(c) Surface energy, E_S = a_SA^2/3

This is incorrect statement.

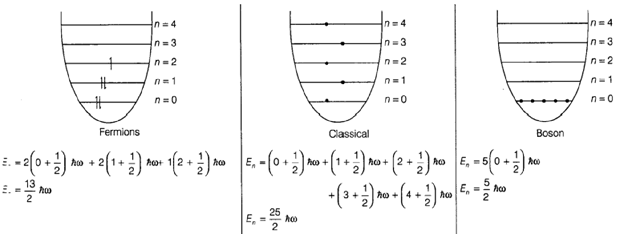
33. Consider a one-dimensional harmonic oscillator of angular frequency 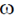. If 5 identical particles occupy the energy levels of this oscillator at zero temperature, which of the following statement (s) about their ground state energy E₀ is (are) correct?

(a)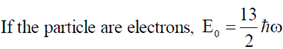

(b)

(c)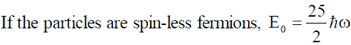

(d)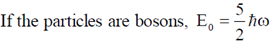

Ans. (a, c, d)

Sol. Energy of one-dimensional harmonic oscillator is

 

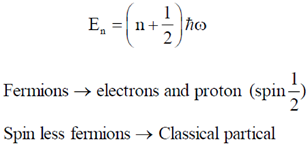
• Fermions Follow Pauli exclusive principle (i.e. no two particles in same state)

• Classical particles (Maxwell-Boltzmaan particles) Atleast one particle in a state

• Bosons All particles can be same (lowest) state.

34. For a point dipole of dipole moment 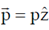located at the origin, which of the following is (are) correct?

(a) The electric field at (0, b, 0) is zero

(b) The work done in moving a charge q from (0, b, 0) to (0, 0, b) is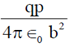

(c) The electrostatic potential at (b, 0, 0) is zero

(d) If a charge q is kept at (0, 0, b) it will exert a force of magnitude 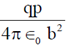on the dipole

Ans. (b, c)

Sol. We know that, electric field at any point due to dipole,

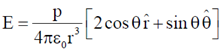
and electric potential,

 

 

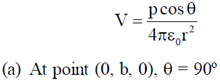
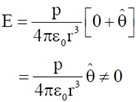
So, option (a) is wrong.

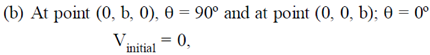
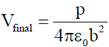
Work done in moving charge q from (0, b, 0) to (0, 0, b),

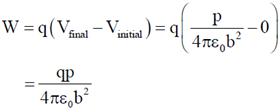
So, option (b) is correct.

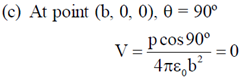
So, option (c) is correct.

(d) There is no force of dipole due to charge, so option (d) is wrong.

35. A dielectric sphere of radius R has constant polarization so that the field inside the sphere is 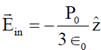. Then, which of the following is (are) correct?

(a)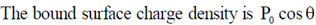

(b)

(c)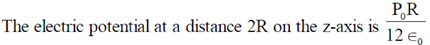

(d) The electric field outside is equivalent to that of a dipole at the origin

Ans. (a, c, d)

Sol. (a) Bound surface charge density,

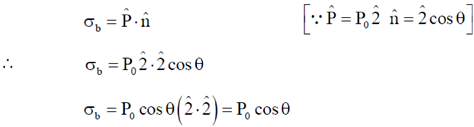
Hence, option (a) is correct.

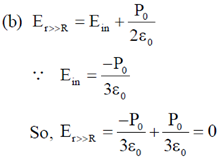
Option (b) is wrong.

(c) Option (c) is correct.

(d) Outside, total charge, q = 0

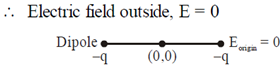
Hence, option (d) is correct.

36. Consider a circular parallel plate capacitor of radius R with separation d between the plates (d << R). The plates are placed symmetrically about the origin. If a sinusoidal voltage V = V₀ sin 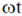is applied between the plates, which of the following statement (s) is (are) true?

(a) The maximum value of the Poynting vector at r = R is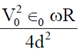

(b) The average energy per cycle flowing out of the capacitor is zero

(c) The magnetic field inside the capacitor is constant

(d) The magnetic field lines inside the capacitor are circular with the curl being independent of r

Ans. (a, b, d)

Sol. (a) Displacement current density,

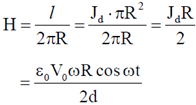
Poynting vector,

S = E × H

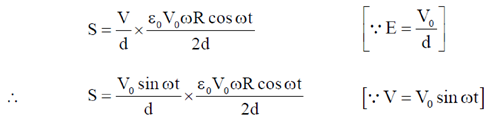
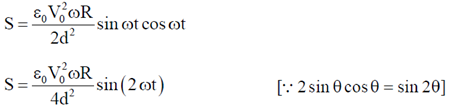
Hence, option (a) is correct.

(b) Average energy flowing out of capacitor is zero, because capacitor stores energy but it is not dissipated.

Hence, option (b) is correct.

(c) Magnetic field inside capacitor varies with distance r,

i.e. B = B(r) = B₀r

Hence, option (c) is wrong.

(d) Magnetic field lines are circular.

Hence, option (d) is correct.

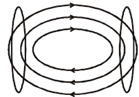
37. The linear mass density of a rod of length l, varies from one end to the other as 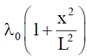, where x is the distance from one end with tensions T₁ and T₂ in them (see figure), and 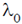is a constant. The rod is suspended from a ceiling by two massless strings. Then, which of the following statement (s) is (are) correct?

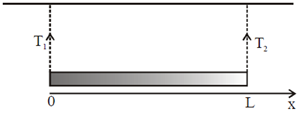
(a)

(b)

(c)

(d)

Ans. (b, c)

Sol.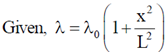

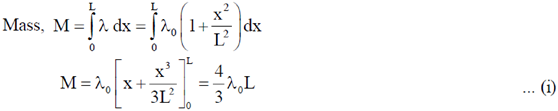
Hence, option (a) is wrong.

Centre of gravity (mass x_CM)

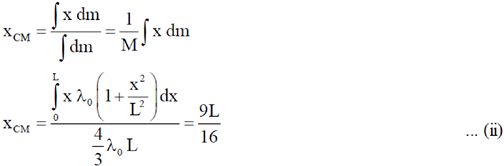
Hence, option (b) is correct.

For equilibrium,

and T₁x_CM = T₂(L – x_CM)

From Eqs. (i) and (ii), we get

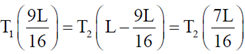
On solving Eqs. (iii) and (iv), we get

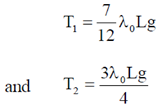
Hence, option (c) is correct and option (d) is wrong.

 

 

 

 

38. An object of mass m with non-zero angular momentum l is moving under the influence of gravitational force of a much larger mass (ignore drag). Which of the following statement (s) is (are) correct?

(a) If the total energy of the system is negative, then the orbit is always circular

(b) The motion of m always occurs in a two dimensional plane

(c) If the total energy of the system is 0, then the orbit is a parabola

(d) If the area of the particle's bound orbit is S, then its time period is 2mS/l

Ans. (b, c, d)

Sol. Trajectory of a satellite based on total energy E.

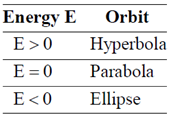
Option (a) is wrong and option (c) is correct.

We know that the motion is under central force is always in a plane.

Hence, option (b) is correct.

 

 

 

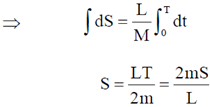
Hence, option (d) is correct.

39. A particle of mass m fixed in space is observed from a frame rotating about its z-axis with angular speed 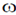. The particle is in the frame's xy plane at a distance R from its origin. If the Coriolis and centrifugal forces on the particle are 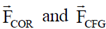, respectively, then (all the symbols have their standard meaning and refer to the rotating frame)

(a)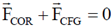

(b)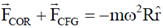

(c)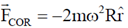

(d)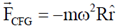

Ans. (b, c)

Sol.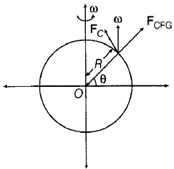

Total force on an object on earth surface due to rotation,

F_i = F_r + F_COR + F_CFG

We know that,

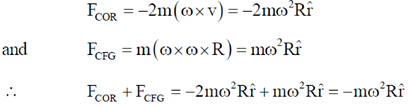
So, option (b, c) are correct.

40. An isolated box is divided into two equal compartments by a partition (see figure). One compartment contains a van der Waals gas while the other compartment is empty. The partition between the two compartments is now removed. After the gas has filled the entire box and equilibrium has been achieved, which of the following statement (s) is (are) correct?

(a) Internal energy of the gas has not changed

(b) Internal energy of the gas has decreased

(c) Temperature of the gas has increased

(d) Temperature of the gas has decreased

Ans. (a, d)

Sol. By the energy relation,

By van der Walls' gas equation,

 

 

 

 

 

 

 

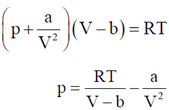
From Eqs. (i) and (ii), we get

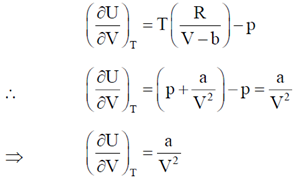
U decrease with volume.

We know that temperature decrease with volume.

So, option (a,d) are correct.

41. An intrinsic semiconductor of band gap 1.25 eV has an electron concentration 10¹⁰ cm^–3 at 300K. Assume that its band gap is independent of the temperature and that the electron concentration depends only exponentially on the temperature. If the electron concentration at 200K is

Y × 10^N cm^–3 (1 < Y < 10, N = integer), then the value of N is ______________

Ans. (4)

Sol.

Ist condition,

IInd condition,

On dividing Eq. (iii) by Eq. (ii), we get

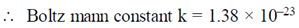
1 eV = 1.6 × 10^–19 J

Put the above values in Eq. (iv), we get

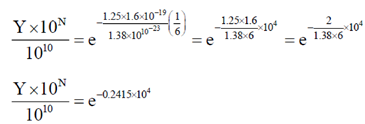
By the solution above equation, we get

42. A particle of unit mass is moving in a one-dimensional potential V(x) = x² – x⁴. The minimum mechanical energy (in the same units as V(x)) above which the motion of the particle cannot be bounded for any given initial condition is _________________

(Specify your answer to two digits after the decimal point)

Ans. (0.25)

Sol. Given, V(x) = x² – x⁴(1 – x²)

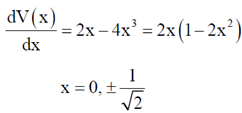
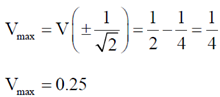
43. In a coaxial cable, the radius of the inner conductor is 2 mm and that of the outer one is 5 mm. The inner conductor is at a potential of 10V, while the outer conductor is grounded. The value of the potential at a distance of 3.5 mm from the axis is __________________

(Specify your answer in volts to two digits after the decimal point)

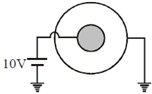
Ans. (2.85)

Sol.

At r = 2 mm, V = 10 V

At r = 5 mm, V = 0

 

 

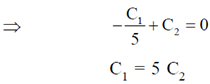
Putting these values from Eqs. (ii) and (iii) into Eq. (i),

 

 

 

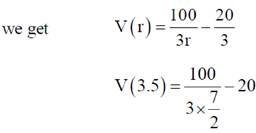
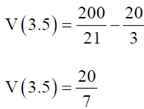
V(3.5) = 2.85

44. Sand falls on a conveyor belt at the rate of 1.5 kg/s. If the belt is moving with a constant speed of 7 m/s, the power needed to keep the conveyor belt running is __________________

(Specify your answer in Watts to two digits after the decimal point)

Ans. (73.5)

Sol. By the definition of force,

By the definition of power,

P = F·v

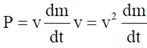
Given that, v = 7 m/s

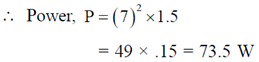
45. A particle of mass m is placed in a three-dimensional cubic box of side a. What is the degeneracy of its energy level with energy ? _________________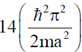

(Express your answer as an integer)

Ans. (6)

Sol. Energy eigenvalues in three-dimensional cubic box,

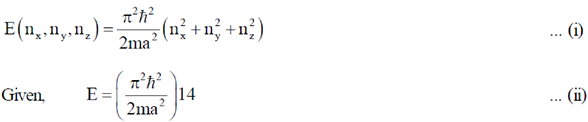
On comparing Eqs. (i) and (ii), we get

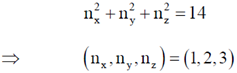
Degeneracy : (n_x, n_y, n_z) = (123)(132)(213)(231)(312)(321)

There is six different energy states, so degeneracy (6 to 6).

46. The wave number of an electromagnetic wave incident on a metal surface is 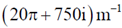inside the metal, where 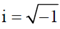. The skin depth of the wave in the metal is __________________

(Specify your answer in mm to two digits after the decimal point)

Ans. (4.6)

Sol. We know that wave vector in conducting medium is

On comparing Eqs. (i) and (ii), we get

 

 

Skin depth is defined as

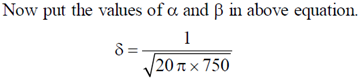
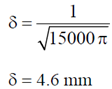
47. Consider a Carnot engine operating between temperatures of 600K and 400K. The engine performs 1000J of work per cycle. The heat (in Joules) extracted per cycle from the high temperature reservoir is _________________

(Specify your answer to two digits after the decimal point)

Ans. (3000)

Sol. Efficiency of Carnot engine is defined as

Q₁ = 3000 J

48. Unpolarized light of intensity I₀ passes through a polarizer P₁. The light coming out of the polarizer falls on a quarter-wave plate with its optical axis at 45º with respect to the polarization axis of P₁ and then passes through another polarizer P₂ with its polarization axis perpendicular to that of P₁. The intensity of the light coming out of P₂ is I. The ratio I₀/I is ________________

(Specify your answer to two digits after the decimal point)

Ans. (4)

Sol.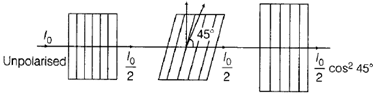

From Malus's law,

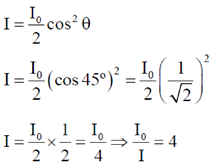
49. An anti-reflection film coating of thickness 0.1 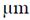is to be deposited on a glass plate for normal incidence of light of wavelength 0.5 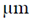. What should be the refractive index of the film? __________

(Specify your answer to two digits after the decimal point)

Ans. (1.25)

Sol. For anti-reflection coating, path difference is given by

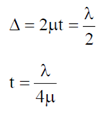
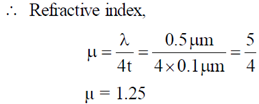
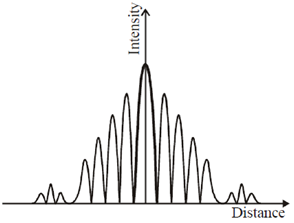
50. Intensity versus distance curve for a double slit diffraction experiment is shown in the figure below. If the width of each of the slits is 0.7 what is the separation between the two slits in micrometers? ___________________

(Specify your answer to two digits after the decimal point)

Ans. (3.50)

Sol. Slits in micrometers

Grating spectra conditions,

 

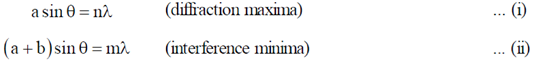
Here, a = slit width

b = length of open portion

(a + b) = grating element or slit separation

From curve n = 1, m = 5

On dividing Eq (ii) by Eq (i), we get

 

 

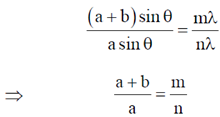
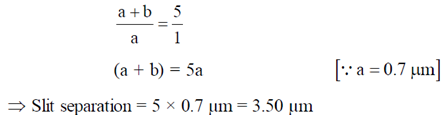
51. The volume integral of the function 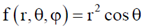over the region

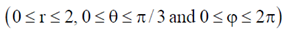is ________________

(Specify your answer to two digits after the decimal point)

Ans. (15.1)

Sol. Volume integral,

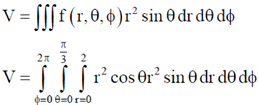
Solution of this integration,

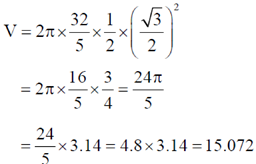
V = 15.1

52.

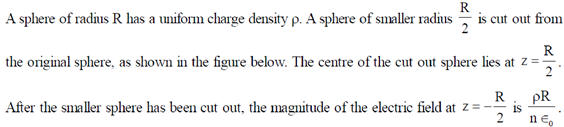
The value of the integer n is ___________________

Ans. (8)

Sol.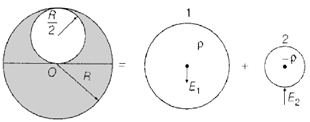

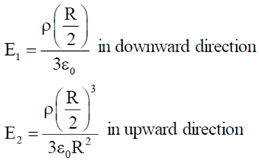
So, net electric field,

E = E₁ – E₂

But given that,

On comparing Eqs. (i) and (ii), we get

n = 8

53. In planar polar co-ordinate, an object's position at time t is given as 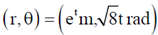. The magnitude of its acceleration in m/s² at t = 0 (to the nearest integer) is _______________

Ans. (9)

Sol.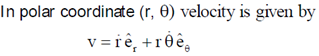

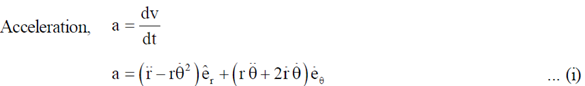
Given,

Now, put the above values in Eq. (i), we get

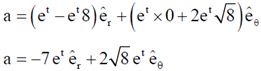
At t = 0,

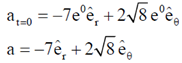
Magnitude of a,

 

 

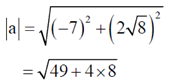
54. Consider two particles moving along the x-axis. In terms of their coordinates x₁ and x₂, their velocities are given as , respectively. When they start moving from their initial locations of x₁(0) = 1 and x₂(0) = –1, the time dependence of both x₁ and x₂ contains a term of the form e^at, where a is a constant. The value of a (an integer) is _______________

Ans. (–2)

Sol.

Now, write Eqs. (i) and (ii) in matrix form,

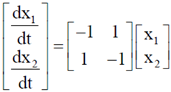
Eigenvalues of matrix,

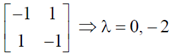
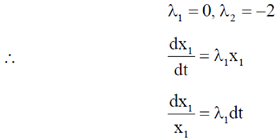
On taking integration both sides, we get

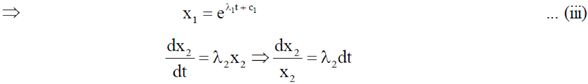
On taking integration both sides, we get

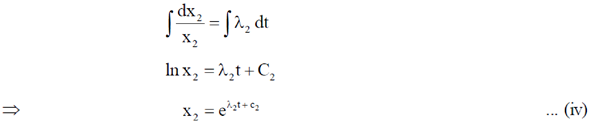
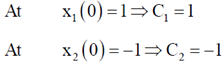
At x₁(t) = e^{0t + 1} [from Eq. (iii)]

x₁(t) = e¹ = e

x₂(t) = e^{–2t – 1} [from Eq. (iv)] ... (v)

x₂(t) = e^{at – 1} ... (vi)

On comparing Eqs. (v) and (vi), we get

a = – 2

 

 

55. For a proton to capture an electron to form a neutron and a neutrino (assumed massless), the electron must have some minimum energy. For such an electron the de-Broglie wavelength in picometers is _____________________

(Specify your answer to two digits after the decimal point)

Ans. (1.08)

Sol.

Rest mass energy = 939.12 – 938.57

= 1.29 MeV

We know that, de-Broglie wavelength,

56.

(Specify your answer to two digits after the decimal point)

Ans. (4.65)

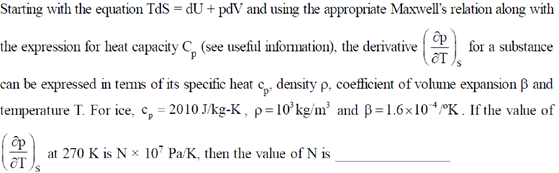
Sol. We know that from Maxwell's equation,

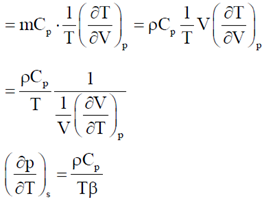
Given, C_p = 2010 J/kg-K

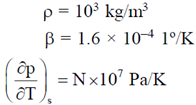
T = 270 K

Now, put above values in Eq. (i), we get

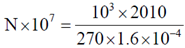
N × 10⁷ = 4.65 × 10⁷

N = 4.65

57. In an electron microscope, electrons are accelerated through a potential difference of 200kV. What is the best possible resolution of the microscope? _________________

(Specify your answer in picometers to two digits after the decimal point)

Ans. (2.74)

Sol. From de-Broglie equation,

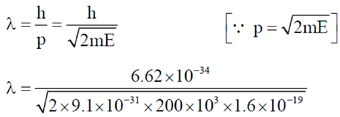
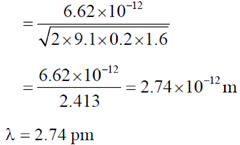
58. Consider the differential equation y" + 2y' + y = 0. If y(0) = 0 and y'(0) = 1, then the value of y(2) is _____________________

(Specify your answer to two digits after the decimal point)

Ans. (0.271)

Sol. (D² + 2D + 1)y = 0

AE

(m² + 2m + 1) = 0

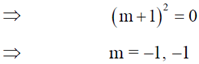
CF

y(x) = (C₁ + C₂x)e^–x

Given conditions,

y(0) = 0

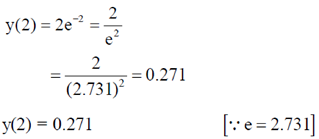
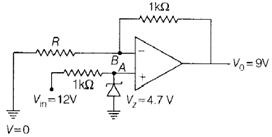
59. An Op-Amp is connected in a circuit with a Zener diode as shown in the figure. The value of resistance R in for obtaining a regulated output V₀ = 9V is ________________

(Specify your answer to two digits after the decimal point)

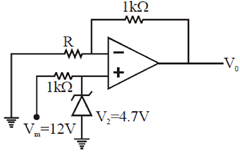
Ans. (1.09)

Sol. V_B = V_A = 4.7 V

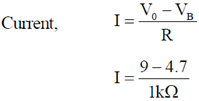
I = 4.3 mA

60. At t = 0, a particle of mass m having velocity v₀ starts moving through a liquid kept in a horizontal tube and experience a drag force 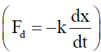. It covers a distance L before coming to rest. If the times taken to cover the distances L/2 and L/4 are t₂ and t₄ respectively, then the ratio t₂/t₄ (ignoring gravity) is _____________________

(Specify your answer to two digits after the decimal point)

Ans. (2.41)

Sol. Equation of motion,

 

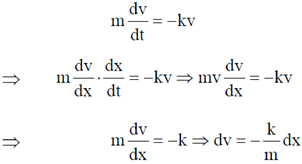
On taking integration both sides, we get

 

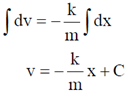
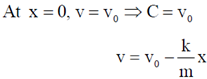
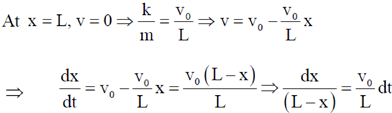
On taking integration both sides, we get