PYQ 2009 - VedPrep

GATE PHYSICS 2009
Previous Year Question Paper with Solution.

1. The value of the contour integral, , for a circle C of radius r with center at the origin is

(a)

(b)

(c)

(d) r

Ans. (a)

Sol.

2. An electrostatic field exists in a given region R. Choose the wrong statement.

(a) Circulation of is zero

(b) can always be expressed as the gradient of a scalar field

(d) The work done in a closed path lying entirely in R is zero

Ans. (c)

Sol. Let any two points on the region A and B.

We know that,

3. The Lagrangian of a free particle in spherical polar co-ordinates is given by . The quantity that is conserved is

(a)

(b)

(c)

(d)

Ans. (c)

Sol.

coordinate is cyclic, therefore canonical momentum is conserved.

4. A conducting loop L of surface area S is moving with a velocity in a magnetic field is a positive constant of suitable dimensions. The emf induced, V_emf , in the loop is given by

(a)

(b)

(c)

(d)

Ans. (d)

Sol. Correct option is (d)

5. The eigenvalues of the matrix are

(a) real and distinct

(b) complex and distinct

(d) real and coinciding

Ans. (b)

Sol.

6. represent the Pauli spin matrices. Which one of the following is not true ?

(a)

(b)

(c)

(d)

Ans. (d)

Sol. The Pauli–spin matrices have following properties

7. Which one of the functions given below represents the bound state eigenfunction of the operator in the region, , with the eigenvalue – 4 ?

(a) A₀e^2x

(b) A₀ cosh 2x

(d) A₀ sinh 2x

Ans. (c)

Sol.

8. Pick the wrong statement

(a) the nuclear force is independent of electric charge

(b) the Yukawa potential is proportinoal to . Where, r is the seperation between two nucleons.

(d) the nucleons interact among each other by the exchange of mesons.

Ans. (b)

Sol. Nuclear force is charge independent.

Yukawa potential is given by

z is atomic number, a is screening parameter

The range of the nuclear force is of the order of two fermi

According to Yukawa Meson Theory, mesons are exchanged during nucteons interaction.

9. If p and q are the position and momentum variables, which one of the following is NOT a canonical transformation ?

(a)

(b)

(d) Q = p and P = – q

Ans. (c)

Sol. For canonical transforma Poisson Bracket {Q, P}_p.q

Therefore, transformation given in option (c) is not a canonical transformation.

10. The Common Mode Rejection Ratio (CMRR) of a differential amplifier using an operational amplifier is 100 dB. The output voltage for a differential input of 200 µV is 2 V. The common mode gain is

(a) 10

(b) 0.1

(d) 10 dB

Ans. (b)

Sol. The common mode rejection ratio,

11. In an insulating solid which one of the following physical phenomena is a consequence of Pauli's exclusion principle ?

(a) Ionic conductivity

(b) Ferromagnetism

(d) Ferroelectricity

Ans. (d)

Sol. For ferromagnetic substance, the specific heat is maximum at T. Thus correct graph of specific heat versus T is expressed as:

Thus, option (d) is not correctly shown.

12. Which one of the following curves gives the solution of the differential equation , where k₁, k₂ and k₃ are positive constant with initial conditions x = 0 at t = 0?

(a)

(b)

(c)

(d)

Ans. (a)

Sol.

Therefore, the solution will be

13. Identify which one is a first order phase transition ?

(a) A liquid to gas transition at its critical temperature

(b) A liquid to gas transition close to its triple point

(d) A metal to superconductor transition in the absence of a magnetic field

Ans. (a)

Sol. The first order phase transition involves the concept of latent heat that is needed when a liquid transform into a gas at its critical temperature.

14. Group- I lists some physical phenomena while Group-II gives some physical parameters. Match the phenomena with the corresponding parameter.

Group I Group II

P. Doppler Broadening 1. Moment of inertia

Q. Natural Broadening 2. Refractive index

R. Rotational spectrum 3. Lifetime of the energy level

S. Total internal reflection 4. Pressure

(a) P-4, Q-3, R-1, S-2

(b) P-3, Q-2, R-1, S-4

(d) P-1, Q-4, R-2, S-3

Ans. (a)

Sol. P : Doppler broadening gives pressure.

Q : Natural broadening gives life time of the energy level

R : Rotational spectrum gives momeiit of inertia

S : Total internal reflection gives refractive index

15. The separation between the first stokes and corresponding anti-stokes lines of the rotational Raman spectrum in terms of the rotational constant, B is :

(a) 2B

(b) 4B

(d) 12B

Ans. (d)

Sol.

The separation between the first stokes and corresponding anti-stokes lines of the rotational Raman spectrum in terms of the rotatbnal constant is 12B.

16. A superconducting ring is cooled in the presence of a magnetic field below its critical temperature (T_C). The total magnetic flux that passes through the ring is

(a) zero

(b)

(c)

(d)

Ans. (b)

Sol. In superconductor, the magnetic flux gets quantised in the units of

17. In a cubic crystal, atoms of mass M₁ lie on one set of planes and atoms of mass M₂ lie on planes interleaved between those of the first set. If C is the force constant between nearest neighbour planes, the frequency of lattice vibrations for the optical phonon branch with wavevector k = 0 is

(a)

(b)

(c)

(d) zero

Ans. (a)

Sol. For optical phonon branch, frequency of lattice vibration is given by

18. In the quark model which one of the following represents a proton?

(a) udd

(b) uud

(c)

(d)

Ans. (b)

Sol. Proton is represented as 'uud'

Since, it has charge and it has Baryon Number

and it has strangeness Number S =(0 + 0 + 0) = 0

19. The circuit shown below:

(a) is a common-emitter amplifier

(b) uses a pnp transistor

(d) has a voltage gain less than one

Ans. (d)

Sol. From figure

Input is base

Output is emitter

A_v; voltage gain of common collector is < 1

A_v(ideally) = 1

CC configurationb is also referred as emitter follower.

20. Consider a nucleus with N neutrons and Z protons. If mp, mn and BE represents the mass of the proton, the mass of the neutron and the binding energy of the nucleus respectively and c is the velocity of light in free space, the mass of the nucleus is given by

(a) Nmn + Zmp

(b) Nmp + Zmn

(c)

(d)

Ans. (c)

Sol. Since, BE = = (mass of total protons + niass of total neutrons-mass of nucleus) × c²

21. The magnetic field (in Am^–1) inside a long solid cylindrical conductor of radius a = 0.1 m, is

. What is the total current (in A) in the conductor ?

(a)

(b)

(c)

(d)

Ans. (b)

Sol.

22. Which one of the following current densities, can generate the magnetic vector potential ?

(a)

(b)

(c)

(d)

Ans. (b)

Sol. We know that.

23. The value of the integral , where the contour C is the circle is

(a)

(b)

(c)

(d)

Ans. (c)

Sol.

24. In a non-conducting medium characterized by and conductivity , the electric field (in V m^–1) is given by . The magnetic field, , is given by

(a)

(b)

(c)

(d)

Ans. (c)

Sol. We know the relation between is

25. A cylindrical rod of length L and radius r, made of an inhomogeneous dielectric, is placed with its axis along the z-direction with one end at the origin as shown below.

If the rod carries a polarization, , the volume bound charge inside the dielectric is

(a) zero

(b)

(c)

(d)

Ans. (c)

Sol. Given : Polarization,

The bound volume charge density,

Therefore, bound volume charge inside the dielectric

26. Let , where is the Levi-Civita density, defined to be zero if two of the indices coincide and +1 and –1 depending on whether ijk is even or odd permutation of 1, 2, 3. Then is equal to

(a) 2a₃

(b) –2a₃

(d) –a₃

Ans. (a)

Sol.

27. The dependence of the magnetic susceptibility of a material with temperature (T) can be represented by is the Curie-Weiss temperature. The plot of magnetic susceptibility versus temperature is sketched in the figure, as curves P, Q and R with curve Q having . Which one of the following statements is correct ?

(a) Curve R represents a paramagnet and Q a ferromagnet

(b) Curve Q represents a ferromagnet and P an antiferromagnet

(d) Curve R represents an antiferromagnet and Q a ferromagnet

Ans. (c)

Sol. From the given figure, it is clear that curve P, Q and R represents versus T graphs for ferromagnetic, paramagnetic and antiferromagnetic materials respectively.

28. The dielectric constant of a material at optical frequencies is mainly due to

(a) ionic polarizability

(b) electronic polarizability

(d) ionic and dipolar polarizability

Ans. (b)

Sol. Optical frequency range 390 THz to 770 THz

In this range dielectric constant of a material due to electronic poiarizability.

29. An electron of wavevector , velocity and effective mass m_e is removed from a filled energy band. The resulting hole has wavevector , velocity , and effective mass m_h. Which one of the following statements is correct ?

(a)

(b)

(c)

(d)

Ans. (d)

Sol.

These relations are obtained by using mass and momentum conservation laws.

30. In a diatomic molecule, the internuclear separation of the ground and first excited electronic state are the same as shown in the figure. If the molecule is initially in the lowest vibrational state of the ground state, then the absorption spectrum will appear as

(a)

(b)

(c)

(d)

Ans. (a)

Sol. For diatomic molecule, the internuclear separation of the ground and first excited state are the same. The absorption spectrum will appear for the transition from lowest vibrational state ofthe ground state will appear as shown in figure:

31. Five energy levels of a system including the ground state are shown below. Their lifetimes and the allowed electric dipole transitions are also marked.

Which one of the following transitions is the most suitable for a continuous wave (CW) laser?

(a)

(b)

(c)

(d)

Ans. (b)

Sol. In all the transitions given above, the transition from is the most suitable for a continuous wave (cw) laser because the state 2 is a metastable state with highest life time of 10^–6 sec.

32. 32. Assuming the mean life time of a muon (in its rest frame) to be 2 × 10^–6 s, its life time in the laboratory frame, when it is moving with a velocity 0.95 c is

(a) 6.4 × 10^–6 s

(b) 0.62 × 10^–6 s

(d) 0.19 × 10^–6 s

Ans. (a)

Sol.

33. Cesium has a nuclear spin of 7/2. The hyperfine spectrum of the D lines of the Cesium atom will consist of

(a) 10 lines

(b) 4 lines

(d) 14 lines

Ans. (a)

Sol. The nuclear spin of Cesium (I)=7/2

For D-lines of the Cesium atom, the transitions will be from ²P_3/2²S_1/2 and ²P_1/2²S_1/2.

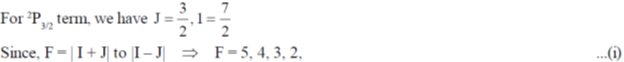
Now, for hyperfine spectrum, the lines are shown with selection rule

Thus, these are total 10 lines in the hyperfine spectrum.

34. The probability that an energy level at a temperature T is unoccupied by a fermion of chemical potential µ is given by

(a)

(b)

(c)

(d)

Ans. (c)

Sol. The probability of occupied state for fermions is

So probability of unoccupied state is

35. Consider the following expression for the mass of a nucleus with Z protons and A nucleons.

Here, f(A) is a function of A.

y = –4aA

z = acA–1/3 + 4aAA–1

aA and ac are constants of suitable dimensions. For a fixed A, the expression of Z for the most stable nucleus is

(a)

(b)

(c)

(d)

Ans. (c)

Sol.

36. The de-Broglie wavelength of particles of mass m with average momentum p at a temperature T in three dimension is given by

(a)

(b)

(c)

(d)

Ans. (b)

Sol. The energy in 3-D is

The de-Broglie wavelength is

37. Assuming an ideal voltage source, Thevenin's resistance and Thevenin's voltage respectively for the below circuit are

(a)

(b)

(c)

(d)

Ans.

Sol. For calculation of Thevenin voltage (V_TH) or open circuit voltage (V_OC) Remove R_L

For applying the thevenin resitance

Condition {Voltagem-soruce is short-circuit and current-souce is open-circuit}

Equilvalent circuit

38. Let and denote the isospin state with , and of a nuclear respectively. Which one of the following two nuclear state has I = 0, I3 = 0?

(a)

(b)

(c)

(d)

Ans. (c)

Sol.

For I = 0 (singlet), wave function whould be-symmetric. Thus, answer can be form (a) and (c).

For I = 0, I₃ = 0

Thus option is (a) is incorrect.

Thus, this corresponds to J₃ = 0 and it is anti-symmetric wave function.

39. An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9 % of its output voltage in series with the input opposing. If f_L = 20 Hz and f_H = 200 kHz for the amplifier without feedback, then due to the feedback

(a) the gain decreases by 10 times

(b) the output resistance increases by 10 times

(d) the input resistance decreases by 100 times

Ans. (c)

Sol. Given : Gain of Amplifier = 1000

The gain of the amplifier with feedback,

So, the gain decrease by 100 times

In the voltage series feedback, the output resistance is given by

Output resistance decrease by 100 times and input resistance

Input resistance increase by 100 times

40. Pick the correct statement based on the below circuit.

(a) The maximum zener current, I_Z(max), when is 15 mA

(b) The minimum zener current, I_Z(min), when is 5 mA

(d) The power dissipated across the zener when and V_in = 20 V is 100 mW

Ans. (c)

Sol. Check the value of given option

The given circuit zener diode will conduct in reverse bias if

S, I_z = 14 mA

I_Z = 4 mA

. So, V_OC > V_BR. Again, zener diode will conduct in reverse bias.

Here, I_S + I_Z + I_L. So, I_Z = 5 – 1 = 4 mA

Hence invalid Θ I_zmin 5 mA (given)

Firstly check diode is conducting or not.

Since, V_OC > V_BR. Then, zener diode will conduct i.e. V_L = V_Z = 100V

41. The disintegration energy is defined to be the difference in the rest energy between the initial and final states. Consider the following process;

The emitted -particle has a kinetic energy 5.17 MeV. The value of disintegration energy is

(a) 5.26 MeV

(b) 5.17 MeV

(d) 2.59 MeV

Ans. (a)

Sol.

Therefore, equation (i)

42. A classical particle is moving in an external potential field V(x, y, z) which is invariant under the following infinitesimal transformations

where R_Z is the matrix corresponding to rotation about the z-axis. The conserved quantities are (the symbols have their usual meaning)

(a) p_x, p_z, L_z

(b) p_x, p_y, L_z, E

(d) p_y, p_z, L_x, E

Ans. (b)

Sol.

are translation, invariance of lagrangian under translation leads to conservation of linear momentum in that direction therefore, p_x and p_y are conserved.

Invariance of Lagrangian under rotation leads to conservation of angular momentum along that axis. Therefore, L is also conserved.

Since potential doesn't explicitly depend on time and is also independent of velocity therefore energy is conserved.

43. The spin function of a free particle in the basis in which S_z is diagonal, can be written as with eigenvalues , respectively. In the given basis, the normalized eigenfunction of S_y with eigenvalue

(a)

(b)

(c)

(d)

Ans. (d)

Sol.

Eigenvalue equation of S_y corresponding to

Assuming x₂ = 1, x₁ = 1

Therefore, the normalized eigen function of S_y corresponding to eigenvalue

44. and represent two physical characteristics of a quantum system. If is Hermitian, then for the product to be Hermitian, it is sufficient that

(a) is Hermitian

(b) is anti-Hermitian

(a) is Hermitian and and commute

(d) is Hermitian and and anti-commute

Ans. (c)

Sol.

= AB (If A and B commutes)

45. Consider the set of vectors in three-dimensional real vector space . Which one of the following statements is true ?

(a) S is not a linearly independent set

(b) S is a basis for

(d) An orthogonal set of vectors cannot be generated from S

Ans. (b)

Sol. Consider the set of vectors in 3-dimensionai real vector space .

S = {(1,1,1), (1,–1,1), (1,1,–1)}

Volume spanned by these vectors

Therefore, S is a set of linearly independent vector and they will form a basis in .

46. For a Fermi gas of N particles in three dimensions at T = 0 K, the Fermi energy, E_F is proportional to

(a) N^2/3

(b) N^3/2

(d) N²

Ans. (a)

Sol. The density of state in 3-D is

The number of particles is

47. The Lagrangian of a diatomic molecule is given by , where m is the mass of each of the atoms and x₁ and x₂ are the displacements of atoms measured from the equilibrium position and k > 0. The normal frequencies are

(a)

(b)

(c)

(d)

Ans. (d)

Sol.

Corresponding matrices are

for frequency of normal modes

48. A particle is in the normalized state which is a superposition of the energy eigenstates and . The average value of energy of the particle in the state is 20 eV.

(a)

(b)

(c)

(d)

Ans. (d)

Sol.

Therefore, the average energy of the particle

Putting this value in above equation

49. The Lagrangian of a particle of mass m moving in one dimension is 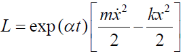, where are positive constant. The equation of motion of the particle is

(a)

(b)

(c)

(d)

Ans. (d)

Sol.

equation of motion

50. Two monochromatic waves having frequencies and and corresonding wavelength and of same polarization, travelling along x-axis are superimposed on each other. The phase velocity and group velocity of the resultant wave are respectively given by

(a)

(b)

(c)

(d)

Ans. (a)

Sol.

Common Data for Questions 51 and 52 :

Consider a two level quantum system with energies and .

51. The Helmholtz free energy of the system is given by

(a)

(b)

(c)

(d)

Ans. (a)

Sol. The partition function is

The Helmholtz free energy is

52. The specific heat of the system is given by

(a)

(b)

(c)

(d)

Ans. (d)

Sol. The internal energy is

The specific heat is

Common Data for Questions 53 and 54 :

A free particle of mass m moves along the x-direction. At t = 0, the normalized wave function of the particle is given by , where is a real constant.

53. The expectation value of the momentum in this state is

(a)

(b)

(c)

(d)

Ans.

Sol. Dimension of wave function in 1-D is (length)^–1/2. But in the given question, dimension of the wave function is (length)^–1/4. Hence question is not correct.

54. The expectation value of the particle energy is

(a)

(b)

(c)

(d)

Ans.

Sol. Dimension of wave function in 1-D is (length)^–1/2. But in the given question dimension of the wave function is (length)^–1/4. Hence question is not correct.

Common Data for Q.55 and Q.56:

Consider the Zeeman splitting of a single electron system for the electric dipole transition.